Types of musical tunings

We are all used to the fact that there are 12 notes in an octave: 7 white keys and 5 black ones. And all the music we hear, from classical to hard rock, is made up of these 12 notes.

Was it always like this? Did music sound like this in the time of Bach, in the Middle Ages or in Antiquity?

Classification convention

Two important facts:

• the first sound recordings in history were made in the second half of the XNUMXth century;
• until the beginning of the XNUMXth century, the fastest speed at which information could be transmitted was the speed of a horse.

Now let’s fast forward a few centuries ago.

Suppose the abbot of a certain monastery (let’s call him Dominic) came up with the idea that it is necessary to sing chants and perform canons everywhere and always the same way. But he cannot call the neighboring monastery and sing his note “A” to them so that they tune theirs. Then the whole brotherhood they make a tuning fork, which reproduces exactly their note “la”. Dominic invites the most musically gifted novice to his place. A novice with a tuning fork in the back pocket of his cassock sits on a horse and for two days and two nights, listening to the whistle of the wind and the clatter of hooves, gallops to a neighboring monastery to unify their musical practice. Of course, the tuning fork bent from the jump, and gives the note “la” inaccurately, and the novice himself, after a long journey, does not remember well whether the notes and intervals sounded like that in his native monastery.

As a result, in two neighboring monasteries, the settings of musical instruments and singing voices turn out to be different.

If we fast forward to the XNUMXth-XNUMXth century, we will find that even notation did not exist then, that is, there were no such notations on paper by which anyone could unambiguously determine what to sing or play. The notation in that era was non-mental, the movement of the melody was indicated only approximately. Then, even if our unlucky Dominic sent a whole choir to a neighboring monastery for a symposium on the exchange of musical experience, it would not be possible to record this experience, and after some time all the harmonies would change in one direction or another.

Is it possible, with such confusion, to speak of any musical structures in that era? Oddly enough, it’s possible.

Pythagorean system

When people began to use the first stringed musical instruments, they discovered interesting patterns.

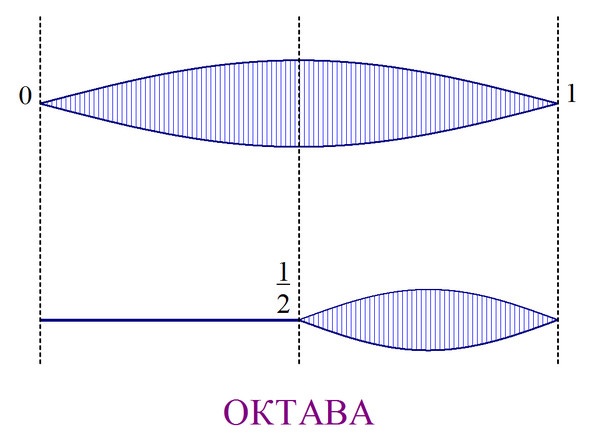
If you divide the length of the string in half, then the sound that it makes is very harmoniously combined with the sound of the whole string. Much later, this interval (the combination of two such sounds) was called octave (pic 1).

Many consider the fifth to be the next harmonious combination. But apparently this was not the case in history. It is much easier to find another harmonious combination. To do this, you just need to divide the string not into 2, but into 3 parts (Fig. 2).

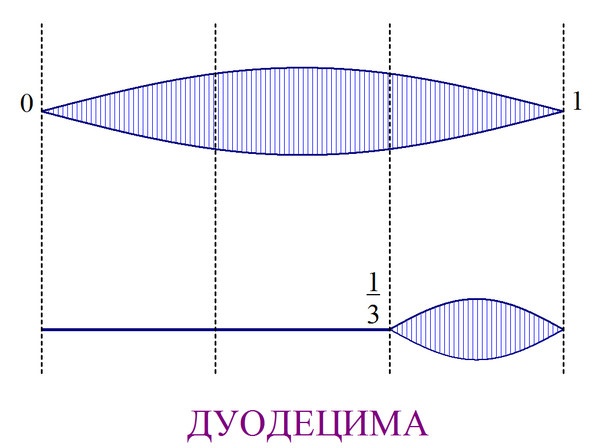
This ratio is now known to us as duodecima  (composite interval).

Now we have not just two new sounds – octave and duodecimal – now we have two ways to get more and more new sounds. It’s dividing by 2 and 3.

We can take, for example, a duodecimal sound (i.e. 1/3 of the string) and divide this part of the string already. If we divide it by 2 (we get 1/6 of the original string), then there will be a sound that is an octave higher than the duodecimal. If we divide by 3, we get a sound that is duodecimal from duodecimal.

You can not only divide the string, but also go in the opposite direction. If the length of the string is increased by 2 times, then we get a sound an octave lower; if you increase by 3 times, then duodecima is lower.

By the way, if the duodecimal sound is lowered by one octave, that is. increase the length by 2 times (we get 2/3 of the original string length), then we will get the same fifth (Fig. 3).

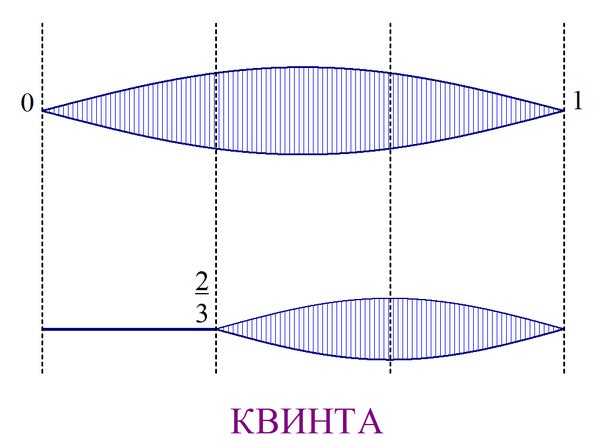
As you can see, a fifth is an interval derived from an octave and a duodecim.

Usually, the first who guessed to use the steps of dividing by 2 and by 3 to build notes is called Pythagoras. Whether this is actually the case is quite difficult to say. And Pythagoras himself is an almost mythical person. The earliest written accounts of his work that we know of were written 200 years after his death. Yes, and it is quite possible to assume that musicians before Pythagoras used these principles, simply did not formulate (or did not write down) them. These principles are universal, dictated by the laws of nature, and if the musicians of the early centuries strove for harmony, they could not bypass them.

Let’s see what kind of notes we get by walking in twos or threes.

If we divide (or multiply) the length of a string by 2, then we will always get a note that is an octave higher (or lower). Notes that differ by an octave are called the same, we can say that we will not get “new” notes in this way.

The situation is quite different with division by 3. Let’s take “do” as the initial note and see where the steps in triplets lead us.

We put it on the axis duodecim for duodecimo (fig. 4).

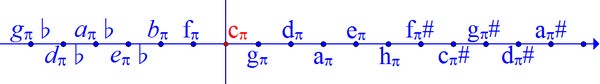
You can read more about the Latin names of notes here. The index π at the bottom of the note means that these are notes of the Pythagorean scale, so it will be easier for us to distinguish them from the notes of other scales.

As you can see, it was in the Pythagorean system that the prototypes of all the notes that we use today appeared. And not only music.

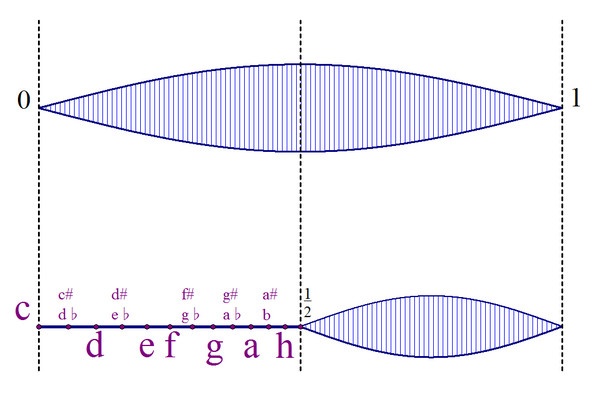
If we take the 5 notes nearest to “do” (from “fa” to “la”), we get the so-called pentatonic – the interval system, which is widely used to this day. The next 7 notes (from “fa” to “si”) will give diatonic. It is these notes that are now located on the white keys of the piano.

The situation with black keys is a little more complicated. Now there is only one key between “do” and “re”, and depending on the circumstances, it is called either C-sharp or D-flat. In the Pythagorean system, C-sharp and D-flat were two different notes and could not be placed on the same key.

natural tuning

What made people change the Pythagorean system to natural? Oddly enough, it is a third.

In the Pythagorean tuning, the major third (for example, the interval do-mi) is rather dissonant. In Fig. 4, we see that in order to get from the note “do” to the note “mi”, we need to take 4 duodecimal steps, divide the string length by 4 3 times. It is not surprising that two such sounds will have little in common, little consonance , that is, consonance.

But very close to the Pythagorean third there is a natural third, which sounds much more consonant.

Pythagorean third

Natural third

Choir singers, when this interval appeared, reflexively took a more consonant natural third.

To get a natural third on a string, you need to divide its length by 5, and then lower the resulting sound by 2 octaves, so the length of the string will be 4/5 (Fig. 5).

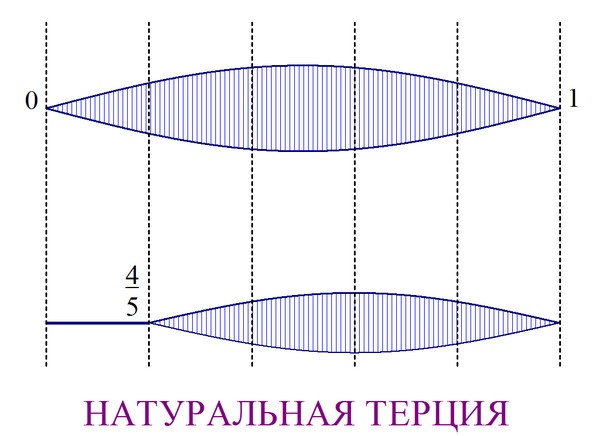
As you can see, the division of the string into 5 parts appeared, which was not in the Pythagorean system. That is why a natural third is impossible in the Pythagorean system.

Such a simple replacement led to a revision of the entire system. Following the third, all intervals except prima, seconds, fourths and fifths changed their sound. Formed natural (sometimes it is called чистый) structure. It turned out to be more consonantal than Pythagorean, but that’s not the only thing.

The main thing that has come to music with natural tuning is tonality. Major and minor (both as chords and as keys) became possible only in natural tuning. That is, formally, a major triad can also be assembled from the notes of the Pythagorean system, but it will not have the quality that allows you to organize the tonality in the Pythagorean system. It is no coincidence that in ancient music the dominant warehouse was monody. Monody is not just monophonic singing, in a sense it can be said that it is monophony, which denies even the possibility of harmonic accompaniment.

There is no point in explaining the meaning of major and minor to musicians.

For non-musicians, the following experiment can be suggested. Include any classical piece from the Viennese classics to the middle of the 95th century. With a probability of 99,9% it will be either in the major or in the minor. Turn on modern popular music. It will be in a major or minor with a probability of XNUMX%.

Tempered scale

There have been many attempts at temperament. Generally speaking, temperament is any deviation of an interval from pure (natural or Pythagorean).

The most successful option was equal temperament (RTS), when the octave was simply divided into 12 “equal” intervals. “Equality” here is understood as follows: each next note is the same number of times higher than the previous one. And having raised the note 12 times, we must come to a pure octave.

Having solved such a problem, we get a 12-note equal temperament (or RTS-12).

But why was temperament needed at all?

The fact is that if in a natural tuning (namely, it was replaced by an evenly tempered one) to change the tonic – the sound from which we “count” the tonality – for example, from the note “do” to the note “re”, then all interval relationships will be violated. This is the Achilles’ heel of all clean tunings, and the only way to fix this is to make all the intervals a little off, but equal to each other. Then when you move to a different key, in fact, nothing will change.

The tempered system has other advantages. For example, it can play music, both written for the natural scale, and for the Pythagorean.

Of the minuses, the most obvious is that all intervals except the octave in this system are false. Of course, the human ear is not an ideal device either. If the falsehood is microscopic, then we can simply not notice it. But the same tempered third is quite far from the natural one.

Natural third

Tempered third

Are there any ways out of this situation? Can this system be improved?

What’s next?

Let’s go back to our Dominic first. Can we say that in the era before sound recording there were some fixed musical tunings?

Our reasoning shows that even if the note “la” shifts, then all constructions (dividing the string into 2, 3 and 5 parts) will remain the same. This means that the systems will essentially turn out to be the same. Of course, one monastery can use the Pythagorean third in its practice, and the second – the natural one, but by determining the method of its construction, we will be able to unambiguously determine the musical structure, and hence the possibilities that different monasteries will have musically.

So what’s next? The experience of the 12th century shows that the search did not stop at the RTS-12. As a rule, the creation of new tunings is carried out by dividing the octave not into 24, but into a larger number of parts, for example, into 36 or XNUMX. This method is very mechanistic and unproductive. We have seen that the constructions begin in the area of ​​simple division of the string, that is, they are connected with the laws of physics, with the vibrations of this same string. Only at the very end of the constructions, the received notes were replaced with comfortable tempered ones. If, however, we temper before we build something in simple proportions, then the question arises: what are we tempering, from which notes do we deviate?

But there is also good news. If in order to rebuild the organ from the note “do” to the note “re”, you would have to twist hundreds of pipes and tubes, now, to rebuild the synthesizer, just press one button. This means that we don’t actually have to play in slightly out of tune temperaments, we can use pure ratios and change them the second the need arises.

But what if we want to play not on electronic musical instruments, but on “analog” ones? Is it possible to build new harmonic systems, use some other principle, instead of the mechanistic division of the octave?

Of course, you can, but this topic is so extensive that we will return to it another time.

Author – Roman Oleinikov

The author expresses his gratitude to the composer Ivan Soshinsky for the provided audio materials