Tact |

German Takt, from lat. tactus – touch

Since the 17th century, the basic unit of meter in music, a section of a piece of music that begins with a strong metrical accent. In musical notation, T. are distinguished by vertical lines standing in front of these accents – bar lines. Historically, T. comes from the accompanying bunks. music of the dance character of uniform beats, the intervals between which are close to the inter-beat intervals of a normal pulse, most accurately estimated in magnitude by direct perception. In mensural music, such a primitive “beating T.” gave nature. a measure of note durations (Latin mensura, hence Italian misura and French mesure, meaning T.). In ars antiqua, longa corresponded to this measure; later in connection with the introduction to polyphonic. the music of smaller note durations, the absolute value of which increased, the role of the unit of measurement passes to brevis; in the 16th century, when the term tactus comes into use, it is equated with the normal size of the semibrevis. Since increases and decreases (“proportions”) could change the duration of notes compared to their normal value (integer valor), along with T. alla semibreve there were T. alla breve (due to halving, brevis was equated to the normal value of semibrevis) and alla minima (when doubled). In the 17th century, when T. was being formed in the modern. sense, semibrevis, which has become a “whole note”, remains a unit corresponding to the value of normal T.; a further increase in its duration, however, is associated with the stretching of the very T., to-ry loses the value of the definition. measures of time. New T. is usually divided by weaker accents into shares (typically 4) or counting times (German Zdhlzeiten), on average, approximately corresponding in duration to mensural T., but b. hours, denoted as quarters of a whole note (=semiminima).

T.’s transformation from a counting unit into a group of counting units (Gruppentakt, in the terminology of H. Schunemann) and the change of modern mensural notation marked the emergence of a new rhythm, which was associated with the separation of music from related arts, the development of instr. music and instr. escort to wok. music and a radical change in music. language. Wed-century. polyphonic thinking gave way to chordal, which found external. expression in the notation in the form of a score, which supplanted in the 17th century. old way of writing otd. voices, and in the emergence in the same 17th century. continuous accompaniment – basso continuo. This accompaniment clearly reveals the double articulation characteristic of new music; along with melodic articulation appears articulation into segments filled with definitions. harmony, which begin at strong moments, often coinciding with the endings of parts of the melody. These accents are regulated by the new music. meter – T., which does not dismember music, but, like a continuous bass, articulates it. Metric signifier. the bar line (sporadically found in organizational tablature from the 14th century, but came into general use in the 17th century) does not indicate a stop or pause (as the boundary of a line of verse), but only a metrical line. accent (i.e., the normal place of the accent, with which, as in accent-type verses, the real accent may not coincide). Unlike all types of verse meter (both associated with music and accent sizes separated from it, where the number of stresses always serves to determine the measure of a verse or line), in specifically muses. In meter, the norm refers only to accentuation and does not determine the size of phrases and periods. But metric. accentuation in music is more complicated than in poetry: instead of a simple opposition of metrically stressed (strong) and non-stressed (weak) syllables, T. is formed by a sequence of stresses that differ in strength. In the 4-beat T., the 1st share is heavily stressed, the 3rd is relatively strong, and the 2nd and 4th are weak. Such a sequence of stresses can be perceived regardless of whether the beats conventionally taken as equal are really equal, or whether this equality is violated by all kinds of agogic. deviations, accelerations, decelerations, fermats, etc. The differences between the shares are expressed not so much in absolute loudness, but in the direction of its changes: for strong shares, advantages are characteristic. a strong start followed by a decrease in volume, for weak beats – on the contrary, an increase in volume (and voltage).

The accent scheme of T. is the norm, with which the real accentuation must be correlated, but the edge may not be realized in sound. The preservation of this scheme in the representation is facilitated by its simplicity, in particular, the even division of note values. In the ratio-based mensural rhythm, juxtapositions of unequal values ​​(1 : 2) are preferred, and therefore larger note values ​​in their “perfect” form are equal to 3 smaller ones. The growing importance of the “imperfect” division of notes into 2 equal parts (starting from the 14th century) allows us to consider this era as a transitional one from modal rhythm (see Modus), or mensural in its pure form, to clock, where all the main. note durations are formed by dividing a whole note into halves, quarters, eighths, sixteenths, etc. The “square” 4-beat structure, with which quarters determine the tempo of music, characterizes the main. type T., “usual size” (English common time), the designation to-rogo (C) in mensural notation indicated tempus imperfectum (brevis = 2 semibreves, in contrast to , denoting tempus perfectum) and prolatio minor (absence of a dot, in contrast to и , indicated that semibrevis is 2, not 3 minimae). Vertical bar through size notation (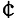), indicating a halving of all durations and equating brevis to the normal value of semibrevis, began to designate T. alla breve, in which, with a 4-beat division, the tempo unit became And not . Such a tempo unit is the main. not only a sign of “big alla breve” (4/2), but also much more common “small alla breve” (2/2), i.e. 2-lobed T., the duration of which is no longer equal to brevis, but whole note (as in C time signature). Designations of other sizes of T. in the form of fractions of the main. sizes also come from the mensural designations of proportions, which, however, have completely changed their meaning. In mensural notation, proportions change the duration of notes without changing the value of time, the unit of time; 3/2, for example, means that 3 notes are equal in duration to two of the same notes of normal size (in modern notation, this is indicated by a triplet –

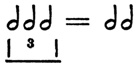
with the difference that the mensural designation is not related to accentuation and does not single out the 1st note of the group as a strong one). Clock notation 3/2 compared to T. 2/2 (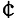) does not change the value of note durations, but increases T. by one and a half times.

As a rule, in a fraction denoting the size of T., the numerator indicates the number of shares, and the denominator indicates their musical value, but there are creatures from this rule. exceptions. According to the number of shares, usually distinguish T. simple with one strong tense (2- and 3-part) and complex, consisting of two or more simple ones, with Ch. accent (strong tense) in the first of them and secondary (relatively strong tenses) in the rest. If these parts are equal, T. called. symmetrical (complex – in a narrower sense), if unequal – asymmetrical or mixed. Complex (symmetry.) T. include 4-, 6-, 9- and 12-beat, mixed – 5-, 7-beat, etc. In this classification, the denominator of the clock designation is not taken into account at all, for example. T. 3/3, 1/3, 2/3, 4/3, 8/3 are classified as 16-part sizes. The difference, obviously, lies not in the duration of the measure beat (for L. Beethoven, the slow part in the 3/8 time can be followed by the fast part in the 3/4 time, where the whole T. is shorter than the eighth of the previous tempo), but in its weight (the smaller notes, the lighter they seem). In the 18th century the choice of note value for the beat was usually limited to a quarter (tempo ordinario) and a half (tempo alla breve); in notation of size with a denominator of 8, the numerator was always divided by 3 (3/8, 6/8, 9/8, 12/8) and did not indicate the number of bases. shares that determine the pace, and their ext. division by 3 (instead of the normal even division). The bipartiteness of T. 6/8 clearly appears in comparisons (simultaneous or successive) with T. 2/4: while maintaining the same tempo, usually

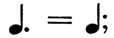
; 9/8 and 12/8 are 3- and 4-beat T. (in classical music, the number of beats in T. does not exceed 4). In 3/8 time, the entire T. (like the mensural T.) often acts as a tempo unit and, therefore, it must be recognized as monolithic (in 3 it is usually conducted at slow tempos, in which the conductor’s gestures do not correspond to the main shares, but to their subdivisions). The same numerators with a denominator of 4 can indicate a triplets division in tempo alla breve: 6/4 is b. h. not a complex T., but a simple 2-part, triplet version 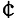. 3/4 can be both 3-part and monopart: in L. Beethoven’s fast tempos, the 1st case is presented in the fugue from the sonata op. 106 ( = 144), the 2nd — in scherzo symphonic ( . = from 96 to 132). Equality T. 3/4 and 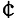in the scherzo of Beethoven’s 3rd and 9th symphonies ( . = = 116) shows that T. 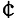could also sometimes be understood as monocot. In the same way, I applied the notation 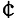A. P. Borodin in the II part of the 2nd symphony; in the score, ed. N. A. Rimsky-Korsakov and A. K. Glazunov it was replaced by 1/1. Monocotyledonous and other simple T. are often grouped into “T. higher order” (sometimes this is indicated by the composer’s remarks, for example “ritmo a tre battute” in the scherzo from Beethoven’s 9th symphony; see Art. Meter).

In the romantic era, the choice of note values ​​for beats becomes more diverse. In Beethoven’s last sonatas, the designations 13/16 and 9/16 indicate that the beat becomes 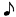., and 6/16 and 12/32 in the 2nd case indicate that in a 3-part T., where the beats are eighth, the triplet division is replaced by an even one (the same change in intralobar pulsation in a 4-part T. can be designated as 8 /8 after 12/8, for example in Liszt’s Preludes). The increasing diversity also applies to the number of shares, which is no longer limited to four. 6/4 can become a real complex T., consisting of both two 3-parts and three 2-parts (with relatively strong 3rd and 5th parts; such T. are found in F. Liszt, S. V. Rachmaninov, I. F. Stravinsky). Mixed (asymmetrical) sizes also appear: 5/4 (the triplet version is 15/8, for example, in Debussy’s Feasts), 7/4, etc. mixed sizes are rare. Sometimes solitary asymmetrical. T. are interspersed among symmetrical ones as their expansion or reduction. B. hours mixed T. represent the union of 2 T. (it is enough to compare 7/4 in Liszt’s Dante Symphony and the alternation of 3/4 and C in his Faust Symphony). Thus, mixed T. tend to turn into phrases, for which the bar line serves as a designation of boundaries, and not strong beats. Such a division into T. is often used when recording music belonging to other rhythms in the clock system. systems, for example. Russian nar. songs (“folk T.” Sokalsky), in themes borrowed by composers from folklore or stylized as it (5/4 by M. I. Glinka, 11/4 by N. A. Rimsky-Korsakov, 9/8

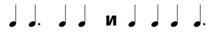
he has it in The Tale of the Invisible City of Kitezh, etc.). Such T.-phrases can be equal in number of shares to the usual simple or complex symmetries. T. (for example, 2/4 in the finale of Tchaikovsky’s 2nd symphony). Outside the Russian music, an example is Chopin’s prelude in c-moll, where each T. is a phrase in which the 1st quarter cannot be considered as a strong time, and the 3rd – as a relatively strong time.

References: Agarkov O., On the adequacy of the perception of a musical meter, in: Musical Art and Science, vol. 1, M., 1970; Kharlap M. G., Clock system in musical rhythm, in collection: Problems of musical rhythm, M., 1978; see also lit. at Art. Meter, Metric.

M. G. Harlap